
Exception: Continuous compounding zero interpolation is used. The sections Zero Bond Discounting Factors and Forward Rates describe how par rates and zero coupon rates are calculated (in the interest calculation method of the yield curve) from interpolated continuous compounding zero bond rates.Ĭalculation of the number of days is always based on the interest calculation method of the yield curve. It is always linear interpolation that is used to calculate the continuous compounding zero bond rates, irrespective of which interpolation procedure is applied. In other words, at the grid points, a yield curve with continuous compounding zero interpolation is identical to the equivalent yield curve without continuous compounding. The equivalent yield curve calculated without continuous compounding is used as the initial yield curve. When continuous compounding zero interpolation is activated, zero bond rates are calculated independently of the yield category in the zero bond discounting factors, using continuous compounding and interest calculation method ACT/365. In this case, there is no calculation of a par rate. For the yield category zero bond yield, first the zero bond rate is interpolated according to the interpolation procedure and from this, the zero bond discounting factor is calculated. The grade school students may use this Linear Interpolation Calculator to generate the work, verify the results or do their homework problems efficiently.Interpolation of interest rates with an interest date different from that of the grid points of a yield curve type when accessing the yield curve.įor the yield category par rate, the par rate is interpolated on the required interest date according to the chosen interpolation procedure of the yield curve type and calculated from the interpolated par rate of the zero coupon and the zero bond discounting factor. For any other combination of coordinates, just supply the coordinates of 2 points and the first coordinate of the required interpolated point and click on the "Generate Work" button. The Linear interpolation work with steps shows the complete step-by-step calculation for finding the value of the $y$-coordinate of an interpolated point, with the value of the x-coordinate of $3$, on the line through points with coordinates $(2, 4)$ and $(7, 8)$. Firstly, we will find the line that contains the points $A$ and $B$.įor a value $x$ in the interval $(x_1,x_2)$, the value $y$ along the non-vertical line through $A$ and $B$ is determined from the slopes

The given points then to find the coordinates of a point between the given points.įor example, given two points $A(x_A,y_A)$ and $B(x_B,y_B)$, as it is shown in the picture below. The procedure for linear interpolation is to find a line that passes through
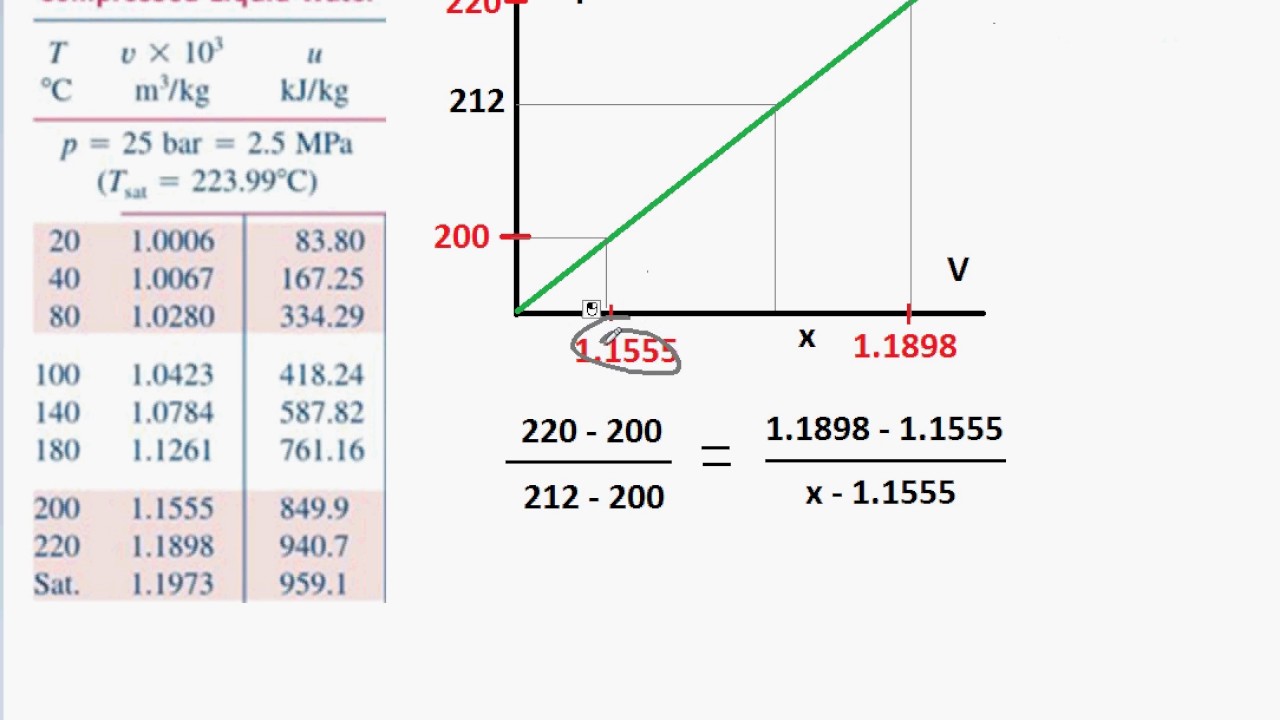
In other words, the linear interpolation is used to fill the gaps in a collection of points.

We can find the equation of a line of best fit, well known as regression line, and use it to minimize the distance of points which represent collection of data to the regression line. Information, especially numerical, appears in all areas of life and science. Note that first coordinates in ordered pairs must be different. Input: Two ordered pairs of real numbers or variables and one real number or variable. This point belongs to the line through points $A$ and $B$.
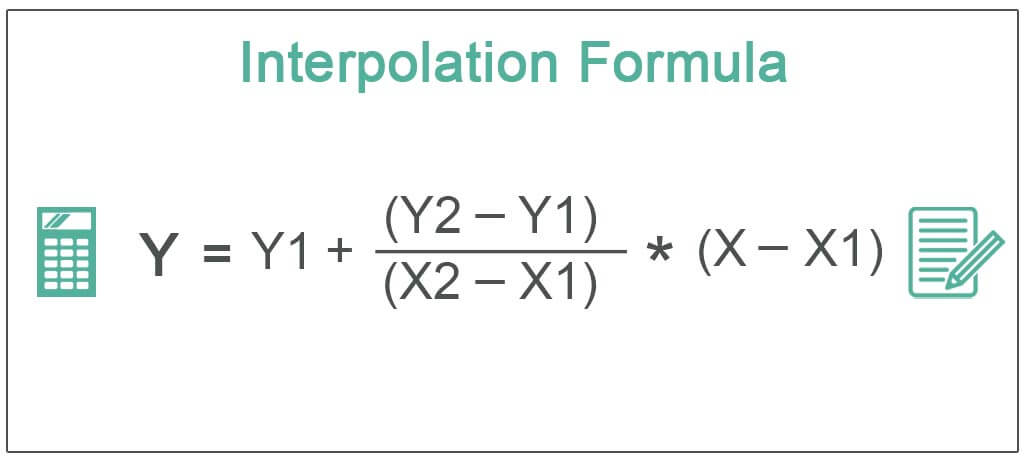
It is necessary to follow the next steps: It's an online Geometry tool requires coordinates of 2 points and $x$-coordinate of the interpolated point in the two-dimensional Cartesian coordinate plane. Linear interpolation calculator uses coordinates of two points $A(x_A, y_A)$ and $B(x_B, y_B)$ and $x$-coordinate of the interpolated point $C$ in the two-dimensional Cartesian coordinate plane and find the $y$-coordinate of the interpolated point $C$.


 0 kommentar(er)
0 kommentar(er)
